SIZE SEGREGATION IN SNOW AVALANCHES

Observations and Experiments

J. MCELWAINE and K. NISHIMURA

Institute of Low Temperature Science, Hokkaido University

Kita-19, Nishi-8, Kita-ku, Sapporo, JAPAN 060

Abstract

In general, well-developed dry snow avalanches consist of at least two stratified layers: snow cloud at the top and dense flowing snow at the bottom [1]. It is well known that the snow cloud sometimes travels faster and farther than the flowing part and may cause serious damage in the runout area. However, since the dense flow often involves most of the mass of the avalanche and is very destructive, understanding its characteristics is of practical significance.

In order to increase our knowledge of avalanche dynamics and to contribute to avalanche zoning and to the design of protection structures, we have carried out natural snow avalanche observations (Shiai Valley, Japan and Ryggfonn, Norway) and also experiments at a ski-jump. In this paper we briefly introduce both approaches and also preliminary results of size segregation experiments.

Measurements

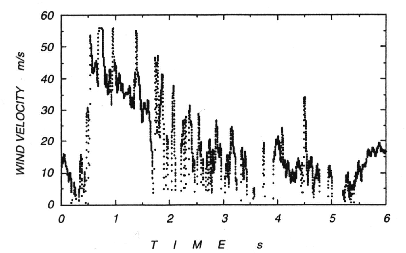
Figure 1: Air velocities in a snow cloud calculated with the recordings of the static pressure depression at the Shiai Valley on January 29 1996.

In the Shiai Valley, a systematic investigation of natural powder snow avalanche has been under way since 1989. In winter, snow usually accumulates to more than 20 m in the valley and the air temperature falls to below $-15\,^0\mbox{C}$ [2]. Shiai Valley runs from an elevation of 1600 m (a.s.l.) to the Kurobe River at an elevation of 600m; its length is about 2000 m, the vertical drop is 1000 m and the average angle of inclination is $33^0$. 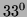At the main observation site, at the midpoint of the avalanche path, instruments were set to measure avalanche impact pressures, wind velocity, wind pressure, atmospheric pressure, temperature and ground vibration. Avalanche movement was recorded with three video cameras. Most of the equipment was installed on two steel mounts of cylinders 0.3 m in diameter and 5 m in height. Data were recorded at a rate of 1 kHz by the data acquisition system in an underground room. Detailed information on the measurement system can be found in Kawada et al.[2] and Nishimura et al.[3].

Figure 2: Velocities of the lower flowing layer of the avalanche calculated by correlating the data from the impact pressure sensors at the Shiai Valley on January 29 1996.

Figure 1 shows the air velocities calculated from the static pressure difference between in an avalanche cloud and in the underground room during the passage of a dry snow avalanche on January 29, 1996. Since the snow cover was observed to be about 2 m deep, it presents the recording at around 3 m above the snow surface. It was the largest avalanche in seven years, and was strong enough to damage the observation tower and to destroy some instruments. The velocity of the snow cloud showed a rapid increase to more than 56 m/s, the limit of measurement with this system. The velocity then declined gradually with periodic fluctuations.

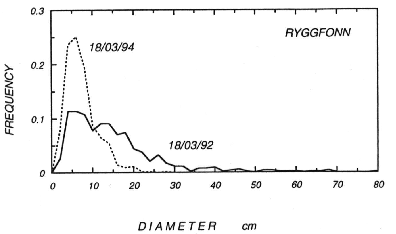
Figure 3: Size distribution of snow blocks observed in avalanche debris in Ryggfonn, Norway. One on March 18, 1994 was a dry snow avalanche and the other one, released on March 18, 1992, was a wet one.

Velocities of the lower flowing layer were also calculated by correlating the data from the impact pressure sensors. The cross-correlation function was calculated at 0.25 s intervals from a time series of impact data to find the average internal snow flow velocity. The velocity was obtained every 0.25 s from a combination of the lag time that gave the highest correlation and the distance between the two measuring points. Further details of the method have been given in Nishimura et al. [3]. Calculated velocities are presented in Figure 2 which shows that the magnitude and the variation of the velocity in the snow cloud are in approximate agreement with the velocity of the dense layer, which suggests a close interaction between the two. Since the interval of calculation here is much coarser than the sampling rate of static pressure depression ( 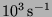), we cannot compare the data directly. However, it is noteworthy, that the velocities of the snow flow also show a periodic change with a dominant frequency of around 1 Hz [5]. Such longitudinal wave-like characteristics were also found by McClung and Schaerer (1985) for both wet and dry avalanches.

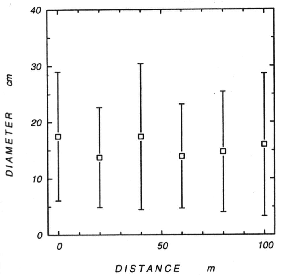
Figure 4: Size distribution of snow blocks along the avalanche path measured in Ryggfonn, Norway on March 18, 1992. Zero on the horizontal axis corresponds to the front of the avalanche debris.

Although precise data are very difficult to obtain, it is known that the flowing layers are usually composed of fluidized snow and a number of snow blocks. Figure 3 shows the size distribution of snow blocks observed in avalanche debris in Ryggfonn, Norway both of which were released with an explosion at 1530 m (a.s.l.) and ran down 1600 m on an avalanche path of 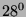$28^0$ mean slope [6-7]. One on March 18, 1994 was a dry snow avalanche and the other one, released on March 18, 1992, was a wet one. In Figure 3 we see the wet one has a wide size distribution and is larger in average (16.0 cm) than the dry one (8.3 cm). In the latter case we also measured the size distribution of snow blocks along the avalanche path every 20m (see Figure 4); zero on the horizontal axis corresponds to the front of the avalanche debris. Although it has been reported in the debris flow that the bigger rocks tend to appear near the front due to segregation processes [8], the snow avalanche in Figure 4 shows a rather uniform distribution.

However, Issler et al. [9] observed that the larger snow blocks existed nearer the surface than the smaller ones by the pit observations of snow avalanche debris. Thus size segregation seem to happen in snow avalanches as well.

In addition it should be also noted that avalanche balloons have been developed as a safety device that can reduce the risk of avalanche burial and are already in practical use. In case of an avalanche, the skier triggers the balloon, which is folded and carried in a specially designed backpack, by releasing pressurized gas from a cartridge. It helps to prevent the victims being buried by increasing his volume. The victim rises through the surrounding smaller snow blocks and particles to the surface of the layer, where, due to the higher velocity at the surface, they move on to the front of the avalanche. Static buoyancy alone cannot explain the effect of the avalanche balloon, since flow densities are typically less than 400 kg/m3 which is about the density of the victim with the inflated balloon. Tschirky and Schweizer[10] carried out the field test and proved its efficiency.

Experiments at a ski jump

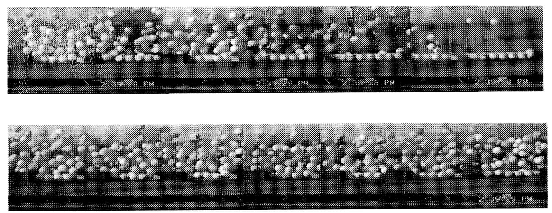
Figure 5: 550,000 ping-pong ball flow along the ski jump.

Perhaps it is right to say that snow avalanches are made up of granular materials. After a dry snow avalanche starts, the snow blocks are broken into smaller lumps or even ice particles. On the other hand, after a wet snow avalanche stops we find a number of snow balls in the debris as shown in Figure 3. Hence, some of the results from studying granular flows can be applied to snow avalanche modeling [11], but unfortunately most of the theories and numerical simulations developed so far appear too simplified to realistically describe the snow avalanches. To investigate granular flows, we carried out inclined chute experiments with snow and ice spheres in a cold laboratory and obtained the profiles of density and velocity as functions of inclination and temperature [12]. However, it was unclear whether the flow reached the steady-state in the 5.4 m long chute. Thus, as a next step, we have started avalanche experiments on the Miyanomori ski jump in Sapporo, because it offers the longest inclined plane under controlled conditions. In winter, natural snow (300 kg maximum) was used. In summer, on the other hand, we have released up to 550,000 ping-pong balls to perform three dimensional granular experiments [13].

The ping-pong balls used in this study were 37.7 mm in diameter and weighted 2.48 g. Since the effect of the air drag acting on such a light ball is fairly large, the flow velocities were expected to arrive at steady state within a short distance. In fact, Nohguchi et al. [14] found in their 22 m long chute experiments that the front velocity of ping-pong ball flow became nearly constant at 10m downstream of the starting point. Furthermore, they concluded with their similarity analysis that the ping-pong flow on the 100 m long slope corresponded to the natural powder snow avalanches which run down for a few kilometer distance [13,14].

In the experiments, up to 500,000 ping-pong balls were stored in a large container set on top of the landing slope. They were released simultaneously by opening the gate of the container. The flow accelerated down along the 150m long and 30 m wide slope, the floor of which was made with an artificial grass and its inclination amounted to 36 deg. from K to P point. The individual movements of the balls and the behavior of the flow were recorded with several video cameras (Figure 5).

Figure 6: Leading edge velocity of the ping-pong ball flow.

Figure 6 shows the leading edge velocity as a function of runout distance when 250,000 ping-pong balls were released from 15 m down the top. The flow accelerated linearly with the distance down the inclined artificial grass floor and its velocity eventually amounted to 15 m/s 65 m down from the starting point. Then the flow kept the velocity almost constant for 30m until the inclination started to decrease; that is a steady granular flow moving at its inherent terminal velocity was obtained. The flow spread out laterally and longitudinally as it moved down the slope (Figure 5) and, after passing the steepest part, the flow came to a stop on the braking track.

Figure 7: Leading edge velocities from K to P point as a function of released ball numbers. The line was derived theoretically by Nohguchi et al. (1996).

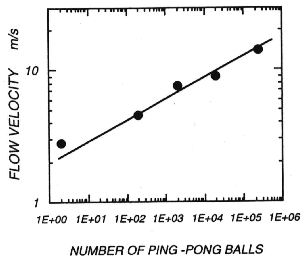
The flow velocities and run out distance strongly depended on the number of released balls. The leading edge velocities measured from K to P point are given in Figure 7 as a function of the number of released balls. The velocity showed a remarkable growth from 2.8 to 15 m/s as the number of ping-pong balls increased from 2 to 300,000. Generally not only air drag but also particle-particle and particle-floor collision act to reduce the velocity. In fact, when two balls were released the velocity was only 2.8 m/s; each ball ran down individually without interaction. This velocity is much less than the free fall velocity of a ping-pong ball, which is $U_t = \ensuremath{9.4\,\mathrm{m/s}}$. However, with an increasing numbers of balls the free fall velocity was reached and surpassed. In fact the largest velocity was 15 m/s which is 1.5 times larger than the free fall velocity.

In the experiments, as the number of balls increased, the head and tail structure became clearer and clearer. When 250,000 balls were released, the thickness of the head was higher than 60 cm which corresponds to about 16 particles diameters. Although the individual balls changed positions rapidly, the leading edge flowed like a consolidated body. Hence, it is reasonable to say that the size of the head gives a strong effect on the flow velocity change listed in Figure 7.

Figure: Ping-pong ball avalanche deposits. Maximum height of the debris was 29 cm. Circles shows the positions where the plastic and polystyrene balls were found.

$\star$	6.8 cm in diameter and 18.1 g in weight
$\bullet$	15 cm and 30.5 g
$\cdot$	10 cm and 10.6 g

Figure 9: Ping-pong flow along the chute of the Shinjo Branch of Snow and Ice Studies, NIED. The flow consists of larger balls (44 mm in diameter and 2.2 g in weight) and ordinary ones (38 mm in diameter and 2.5 g in weight).

In addition to the velocity and particle concentration measurements described above, we have put some particles (balls) with different size and densities from the ping-pong balls in the container in order to look at the segregation process in the flow. Although more experiments and careful analysis will be necessary, it might be noted that a plastic ball, the diameter of which is about twice that of ping-pong ball (6.8 cm in diameter) and the density of which is comparable (18.1 g in weight), appeared at the front part of the debris (Figure 8). Further, aiming at investigating segregation process in granular flows along the chute, we have also carried out the ping-pong flow experiments in the chute of the Shinjo Branch of Snow and Ice Studies, NIED, Japan. The chute has a slope angle of 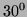$30^0$ and a width of 1 m. One side of the wall consists of glass plate, while the other side is made of wood. Larger ping-pong balls, 44 mm in diameter and 2.2 g in weight are used as well as ordinary ones. All the balls were kept in a container at the top of the chute and released simultaneously. Before each run the ping-pong balls are spread all over the floor to make a rough and uniform surface. Figure 9 shows the flow situation. At this stage no clear findings have been obtained, but we expect that the combination of the above experiments and the simulation of 3-dimensional, inhomogeneous two-phase flows, will make it possible to reveal the segregation process in avalanche flows.

Acknowledgments

The authors gratefully acknowledge all our colleagues who joined our measurements at Shiai Valley and experiments at the ski jump. It is certain the work could not have been completed without their cooperation. These projects were partly supported by Grant-in-Aid for Co-operative Research from the Science Research of the Ministry of education, Science and Culture, Japan.

References

1.

Schaerer, P. A., Friction coefficient and speed of flowing avalanches. International Association of Hydrological Sciences Publication 114 (Symposium at Grindelwald 1974 -- Snow Mechanics), 425-432, 1975.

2.

Kawada, K., K. Nishimura, and N. Maeno, 1989. Experimental studies on a powder snow avalanche, Annals of Glaciology, 13, 129-134.

3.

Nishimura, K., H. Narita, N. Maeno, and K. Kawada, 1989. The internal structure of powder-snow avalanches, Annals of Glaciology, 13, 207-210.

4.

Nishimura, K. and Y. Ito, 1997. Velocity distribution in snow avalanches. Journal of Geophysical Research, Vol. 102, No. B12, 27297-27303.

5.

McClung, D. M. and P. A. Schaerer, 1985. Characteristics of flowing snow and avalanche impact pressures, Annals of Glaciology, 6, 9-14.

6.

Nishimura, K., N. Maeno, F. Sandersen, K. Kristensen, H. Norem, and K. Lied, 1993. Observations of the dynamic structure of snow avalanches, Annals of Glaciology, 18, 313-316.

7.

Nishimura, K., F. Sandersen, K. Kristensen, and K. Lied, 1995. Measurements of powder snow avalanche -Nature-, Surveys in Geophysics, 16, 649-660.

8.

Curry, R. R., 1966. Observation of Alpine mud-flows in the Tenmile range, Central Colorado, Bull. Geol. Soc. Am., Vol. 77, 771-776.

9.

Issler, D., P. Gauer, M. Schaer and S. Keller, 1996. Staublawinenereignisse im Winter 1995: Seewis, Adelboden und Col du Pillon, Interner Bericht Nr. 694.

10.

Tschirky, F and J. Schweizer, Avalanche balloons -preliminary test results, Proceedings of the International Snow Science Workshop, 1996 in Banff, 309-312, 1997.

11.

Savage, S. B., 1983. The flow of granular materials, Chemical Engineering Science, Vol. 38, No.2, 189-195, 1983.

12.

Nishimura, K., K. Kosugi, and M. Nakagawa, 1993. Experiments on ice-sphere flows along an inclined chute, Mechanics of Materials, 16, 205-209.

13.

Nishimura, K., S. Keller, J. McElwaine and Y. Nohguchi, 1998. Ping-pong ball avalanche at a ski jump, Granular Matter, Vol. 1, 51-56.

14.

Nohguchi, Y., K. Nishimura, T. Kobayashi, K. Iwanami, K. Kawashima, Y. Yamada, H. Nakamura, K. Kosugi, O. Abe, A. Sato, Y. Endo, Y. Kominami, and K. Izumi, 1996. Similarity of avalanche experiments by light particles, Proceedings of INTERPRAVENT 1996 -Garmisch-Partenkirchen, Tagungspublikation, Band 2, 147-156.

About this document ...

This document was generated using the LaTeX2HTML translator Version 99.2beta8 (1.42)

Copyright © 1993, 1994, 1995, 1996, Nikos Drakos, Computer Based Learning Unit, University of Leeds.
Copyright © 1997, 1998, 1999, Ross Moore, Mathematics Department, Macquarie University, Sydney.

The command line arguments were:
latex2html -split 0 -image_type gif -notransparent segregation

The translation was initiated by Jim McElwaine on 2000-11-14